Lagrange point

In celestial mechanics, the Lagrange points (/ləˈɡrɑːndʒ/; also Lagrangian points or libration points) are points of equilibrium for small-mass objects under the gravitational influence of two massive orbiting bodies. Mathematically, this involves the solution of the restricted three-body problem.^[1]

Normally, the two massive bodies exert an unbalanced gravitational force at a point, altering the orbit of whatever is at that point. At the Lagrange points, the gravitational forces of the two large bodies and the centrifugal force balance each other.^[2] This can make Lagrange points an excellent location for satellites, as orbit corrections, and hence fuel requirements, needed to maintain the desired orbit are kept at a minimum.

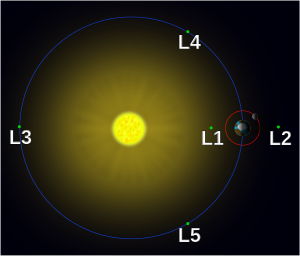
For any combination of two orbital bodies, there are five Lagrange points, L₁ to L₅, all in the orbital plane of the two large bodies. There are five Lagrange points for the Sun–Earth system, and five different Lagrange points for the Earth–Moon system. L₁, L₂, and L₃ are on the line through the centers of the two large bodies, while L₄ and L₅ each act as the third vertex of an equilateral triangle formed with the centers of the two large bodies.

When the mass ratio of the two bodies is large enough, the L₄ and L₅ points are stable points meaning that objects can orbit them, and that they have a tendency to pull objects into them. Several planets have trojan asteroids near their L₄ and L₅ points with respect to the Sun; Jupiter has more than one million of these trojans.

Some Lagrange points are being used for space exploration. Two important Lagrange points in the Sun-Earth system are L₁, between the Sun and Earth, and L₂, on the same line at the opposite side of the Earth; both are well outside the Moon's orbit. Currently, an artificial satellite called the Deep Space Climate Observatory (DSCOVR) is located at L₁ to study solar wind coming toward Earth from the Sun and to monitor Earth's climate, by taking images and sending them back.^[3] The James Webb Space Telescope, a powerful infrared space observatory, is located at L₂.^[4] This allows the satellite's large sunshield to protect the telescope from the light and heat of the Sun, Earth and Moon. The L₁ and L₂ Lagrange points are located about 1,500,000 km (930,000 mi) from earth.

The European Space Agency's earlier Gaia telescope, and its newly launched Euclid, also occupy orbits around L₂. Gaia keeps a tighter Lissajous orbit around L₂, while Euclid follows a halo orbit similar to JWST. Each of the space observatories benefit from being far enough from Earth's shadow to utilize solar panels for power, from not needing much power or propellant for station-keeping, from not being subjected to the Earth's magnetospheric effects, and from having direct line-of-sight to Earth for data transfer.

History

The three collinear Lagrange points (L₁, L₂, L₃) were discovered by the Swiss mathematician Leonhard Euler around 1750, a decade before the Italian-born Joseph-Louis Lagrange discovered the remaining two.^[5]^[6]

In 1772, Lagrange published an "Essay on the three-body problem". In the first chapter he considered the general three-body problem. From that, in the second chapter, he demonstrated two special constant-pattern solutions, the collinear and the equilateral, for any three masses, with circular orbits.^[7]

Lagrange points

The five Lagrange points are labelled and defined as follows:

L₁ point

The L₁ point lies on the line defined between the two large masses M₁ and M₂. It is the point where the gravitational attraction of M₂ and that of M₁ combine to produce an equilibrium. An object that orbits the Sun more closely than Earth would typically have a shorter orbital period than Earth, but that ignores the effect of Earth's gravitational pull. If the object is directly between Earth and the Sun, then Earth's gravity counteracts some of the Sun's pull on the object, increasing the object's orbital period. The closer to Earth the object is, the greater this effect is. At the L₁ point, the object's orbital period becomes exactly equal to Earth's orbital period. L₁ is about 1.5 million kilometers, or 0.01 au, from Earth in the direction of the Sun.^[1]

L₂ point

The L₂ point lies on the line through the two large masses beyond the smaller of the two. Here, the combined gravitational forces of the two large masses balance the centrifugal force on a body at L₂. On the opposite side of Earth from the Sun, the orbital period of an object would normally be greater than Earth's. The extra pull of Earth's gravity decreases the object's orbital period, and at the L₂ point, that orbital period becomes equal to Earth's. Like L₁, L₂ is about 1.5 million kilometers or 0.01 au from Earth (away from the sun). An example of a spacecraft designed to operate near the Earth–Sun L₂ is the James Webb Space Telescope.^[8] Earlier examples include the Wilkinson Microwave Anisotropy Probe and its successor, Planck.

L₃ point

The L₃ point lies on the line defined by the two large masses, beyond the larger of the two. Within the Sun–Earth system, the L₃ point exists on the opposite side of the Sun, a little outside Earth's orbit and slightly farther from the center of the Sun than Earth is. This placement occurs because the Sun is also affected by Earth's gravity and so orbits around the two bodies' barycenter, which is well inside the body of the Sun. An object at Earth's distance from the Sun would have an orbital period of one year if only the Sun's gravity is considered. But an object on the opposite side of the Sun from Earth and directly in line with both "feels" Earth's gravity adding slightly to the Sun's and therefore must orbit a little farther from the barycenter of Earth and Sun in order to have the same 1-year period. It is at the L₃ point that the combined pull of Earth and Sun causes the object to orbit with the same period as Earth, in effect orbiting an Earth+Sun mass with the Earth-Sun barycenter at one focus of its orbit.

L₄ and L₅ points

The L₄ and L₅ points lie at the third vertices of the two equilateral triangles in the plane of orbit whose common base is the line between the centers of the two masses, such that the point lies 60° ahead of (L₄) or behind (L₅) the smaller mass with regard to its orbit around the larger mass.

Stability

The triangular points (L₄ and L₅) are stable equilibria, provided that the ratio of M₁/M₂ is greater than 24.96.^{[note 1]} This is the case for the Sun–Earth system, the Sun–Jupiter system, and, by a smaller margin, the Earth–Moon system. When a body at these points is perturbed, it moves away from the point, but the factor opposite of that which is increased or decreased by the perturbation (either gravity or angular momentum-induced speed) will also increase or decrease, bending the object's path into a stable, kidney bean-shaped orbit around the point (as seen in the corotating frame of reference).^[9]

The points L₁, L₂, and L₃ are positions of unstable equilibrium. Any object orbiting at L₁, L₂, or L₃ will tend to fall out of orbit; it is therefore rare to find natural objects there, and spacecraft inhabiting these areas must employ a small but critical amount of station keeping in order to maintain their position.

Natural objects at Lagrange points

Due to the natural stability of L₄ and L₅, it is common for natural objects to be found orbiting in those Lagrange points of planetary systems. Objects that inhabit those points are generically referred to as 'trojans' or 'trojan asteroids'. The name derives from the names that were given to asteroids discovered orbiting at the Sun–Jupiter L₄ and L₅ points, which were taken from mythological characters appearing in Homer's Iliad, an epic poem set during the Trojan War. Asteroids at the L₄ point, ahead of Jupiter, are named after Greek characters in the Iliad and referred to as the "Greek camp". Those at the L₅ point are named after Trojan characters and referred to as the "Trojan camp". Both camps are considered to be types of trojan bodies.

As the Sun and Jupiter are the two most massive objects in the Solar System, there are more known Sun–Jupiter trojans than for any other pair of bodies. However, smaller numbers of objects are known at the Lagrange points of other orbital systems:

The Sun–Earth L₄ and L₅ points contain interplanetary dust and at least two asteroids, 2010 TK7 and 2020 XL5.^[10]^[11]^[12]
The Earth–Moon L₄ and L₅ points contain concentrations of interplanetary dust, known as Kordylewski clouds.^[13]^[14] Stability at these specific points is greatly complicated by solar gravitational influence.^[15]
The Sun–Neptune L₄ and L₅ points contain several dozen known objects, the Neptune trojans.^[16]
Mars has four accepted Mars trojans: 5261 Eureka, 1999 UJ7, 1998 VF31, and 2007 NS2.
Saturn's moon Tethys has two smaller moons of Saturn in its L₄ and L₅ points, Telesto and Calypso. Another Saturn moon, Dione also has two Lagrange co-orbitals, Helene at its L₄ point and Polydeuces at L₅. The moons wander azimuthally about the Lagrange points, with Polydeuces describing the largest deviations, moving up to 32° away from the Saturn–Dione L₅ point.
One version of the giant impact hypothesis postulates that an object named Theia formed at the Sun–Earth L₄ or L₅ point and crashed into Earth after its orbit destabilized, forming the Moon.^[17]
In binary stars, the Roche lobe has its apex located at L₁; if one of the stars expands past its Roche lobe, then it will lose matter to its companion star, known as Roche lobe overflow.^[18]

Objects which are on horseshoe orbits are sometimes erroneously described as trojans, but do not occupy Lagrange points. Known objects on horseshoe orbits include 3753 Cruithne with Earth, and Saturn's moons Epimetheus and Janus.

Physical and mathematical details

Lagrange points are the constant-pattern solutions of the restricted three-body problem. For example, given two massive bodies in orbits around their common barycenter, there are five positions in space where a third body, of comparatively negligible mass, could be placed so as to maintain its position relative to the two massive bodies. This occurs because the combined gravitational forces of the two massive bodies provide the exact centripetal force required to maintain the circular motion that matches their orbital motion.

Alternatively, when seen in a rotating reference frame that matches the angular velocity of the two co-orbiting bodies, at the Lagrange points the combined gravitational fields of two massive bodies balance the centrifugal pseudo-force, allowing the smaller third body to remain stationary (in this frame) with respect to the first two.

L₁

The location of L₁ is the solution to the following equation, gravitation providing the centripetal force:

{\frac {M_{1}}{(R-r)^{2}}}-{\frac {M_{2}}{r^{2}}}=\left({\frac {M_{1}}{M_{1}+M_{2}}}R-r\right){\frac {M_{1}+M_{2}}{R^{3}}}

where r is the distance of the L₁ point from the smaller object, R is the distance between the two main objects, and M₁ and M₂ are the masses of the large and small object, respectively. The quantity in parentheses on the right is the distance of L₁ from the center of mass. The solution for r is the only real root of the following quintic function

x^{5}+(\mu -3)x^{4}+(3-2\mu )x^{3}-(\mu )x^{2}+(2\mu )x-\mu =0

where

\mu ={\frac {M_{2}}{M_{1}+M_{2}}}

is the mass fraction of M₂ and

x={\frac {r}{R}}

is the normalised distance. If the mass of the smaller object (M₂) is much smaller than the mass of the larger object (M₁) then L₁ and L₂ are at approximately equal distances r from the smaller object, equal to the radius of the Hill sphere, given by:

r\approx R{\sqrt{\frac {\mu }{3}}}

We may also write this as:

{\frac {M_{2}}{r^{3}}}\approx 3{\frac {M_{1}}{R^{3}}}

Since the tidal effect of a body is proportional to its mass divided by the distance cubed, this means that the tidal effect of the smaller body at the L₁ or at the L₂ point is about three times of that body. We may also write:

\rho _{2}\left({\frac {d_{2}}{r}}\right)^{3}\approx 3\rho _{1}\left({\frac {d_{1}}{R}}\right)^{3}

where ρ₁ and ρ₂ are the average densities of the two bodies and d₁ and d₂ are their diameters. The ratio of diameter to distance gives the angle subtended by the body, showing that viewed from these two Lagrange points, the apparent sizes of the two bodies will be similar, especially if the density of the smaller one is about thrice that of the larger, as in the case of the earth and the sun.

This distance can be described as being such that the orbital period, corresponding to a circular orbit with this distance as radius around M₂ in the absence of M₁, is that of M₂ around M₁, divided by √3 ≈ 1.73:

T_{s,M_{2}}(r)={\frac {T_{M_{2},M_{1}}(R)}{\sqrt {3}}}.

L₂

The location of L₂ is the solution to the following equation, gravitation providing the centripetal force:

{\frac {M_{1}}{(R+r)^{2}}}+{\frac {M_{2}}{r^{2}}}=\left({\frac {M_{1}}{M_{1}+M_{2}}}R+r\right){\frac {M_{1}+M_{2}}{R^{3}}}

[1]

[2]

[3]

[4]

[5]

[6]

[7]

[8]

[note 1]

[9]

[10]

[11]

[12]

[13]

[14]

[15]

[16]

[17]

[18]

[19]

History

Lagrange points

L1 point

L2 point

L3 point

L4 and L5 points